可积系统最新进展学术研讨会
Workshop on Recent Advances in Integrable Systems
2017年11月17-18日
澳门十大娱乐平台正规
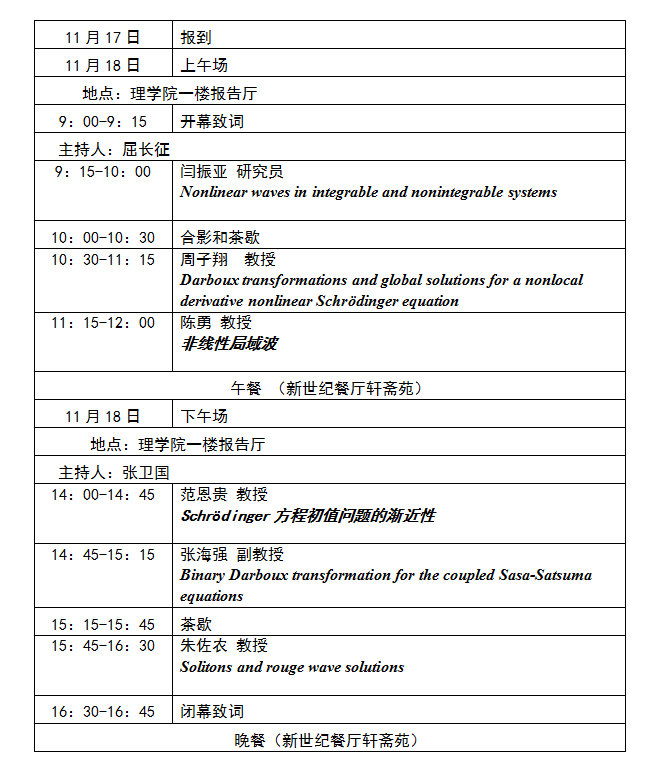
会议日程
报告题目和摘要
Nonlinear waves in integrable and nonintegrable systems
闫振亚
中国科学院数学与系统科学研究院
摘要:Introducing some our work on nonlinear waves in integrable and nonintegrable systems.
报告人简介:闫振亚,大连理工大学博士,中科院数学与系统科学研究院研究员,全国百篇优秀博士论文获得者,荣获中国科学院2008年卢嘉锡青年人才奖。研究方向为复杂非线性波、可积性、符号分析等。
Darboux transformations and global solutions for a nonlocal derivative nonlinear Schrödinger equation
周子翔
复旦大学数学科学学院
摘要:A nonlocal derivative nonlinear Schrodinger equation is discussed. By constructing its Darboux transformations of degree 2n, the explicit expressions of new solutions are derived from zero seed solutions. Usually the derived solutions of this nonlocal equation may have singularities. However, it is shown that the solutions of the nonlocal derivative nonlinear Schrodinger equation can be globally defined and bounded for all (x,t) if the eigenvalues and the parameters characterizing the ratio of the two entries of the solutions of the Lax pair are chosen properly.
报告人简介:周子翔,复旦大学数学科学学院教授,博士生导师,主要研究方向是可积系统,尤其是Darboux变换等相关方法及其在微分几何与物理中的应用。2000年入选教育部跨世纪优秀人才支持计划。
非线性局域波
陈勇
华东师范大学计算机与软件工程学院
摘要:首先介绍局域波的发展概况,然后介绍我们团队近年来关于局域波开展的研究工作。主要在以下三个方面:达布变换;KP约化;双线性算法。以及相应的程序算法进展情况。
报告人简介:陈勇,华东师范大学教授、博士生导师,主要从事非线性数学物理、可积系统、计算机符号计算和程序开发的研究;主持和参与国家自然科学基金面上项目、博士点基金、两次国家基金委重点项目基金、连续两届国家自然科学基金创新群体基金(项目骨干成员)、国家重大科学研究计划项目(973)等项目(骨干科学家)。
Schrodinger方程初值问题解的渐近性
范恩贵
复旦大学数学科学学院
摘要:首先将Schrodinger方程具有快速衰减的初值问题转化为Riemann-Hilbert问题,然后用非线性速降法给出Schrodinger方程初值问题解的渐近性。
报告人简介:范恩贵,复旦大学教授、博士生导师,曾获教育部自然科学二等奖、上海市自然科学二等奖、国际“汤姆森路透卓越研究奖”、复旦大学谷超豪数学奖;主要研究方向是孤立子理论、可积系统、Riemann-Hilbert问题、正交多项式和随机矩阵理论;近年来,连续两届为国家“973”课题成员,并主持国家自然科学基金、上海曙光计划、上海曙光计划跟踪课题等多项研究课题。
Binary Darboux transformation for the coupled Sasa-Satsuma equations
张海强
澳门十大娱乐平台正规
摘要:The binary Darboux transformation method is applied to the coupled Sasa-Satsuma equations, which can be used to describe the propagation dynamics of femtosecond vector solitons in the birefringent fibers with third-order dispersion, self-steepening, and stimulated Raman scattering higher-order effects. An N-fold iterative formula of the resulting binary Darboux transformation is presented in terms of the quasideterminants. Via the simplest case of this formula, a few of illustrative explicit solutions to the coupled Sasa-Satsuma equations are generated from vanishing and non-vanishing backgrounds, which include the breathers, single- and double-hump bright vector solitons, and anti-dark vector solitons.
Solitons and rouge wave solutions
朱佐农
上海交通大学数学科学学院
报告人简介:朱佐农,上海交通大学数学系教授,博士生导师。学术研究领域是数学物理,研究方向是孤立子和可积系统理论,在连续和离散的可积系统的研究上取得若干重要进展。先后主持国家自然科学基金项目、
欢迎各位老师和同学前来参加!

